Spotblog402

1. Blackjack Double Down Rule
2. Blackjack Double Down Odds Genesis Open
3. Double Down Blackjack Meaning
4. Blackjack Double Down Odds Explained
5. Blackjack Double Down Odds Poker
6. Blackjack Odds Sheet
7. Blackjack Double Down Odds Video Poker

By Ion Saliu, Founder of Blackjack Mathematics

Blackjack Double Down Probabilities; Blackjack Double Down Probabilities. This chart shows exact percentages for how often each double-down hand in blackjack will win, push, or lose. The figures quoted are for a 6 deck game where the dealer stands on all 17s. The dealer is assumed to have already peeked under tens and aces and does not have. Therefore, unless you can find a casino that offers a 3:2 payout for single-deck blackjack it is a wise decision to stick to multiple deck games. Single deck blackjack game. Casinos also often enforce disadvantageous rule variations on single-deck and double-deck games in order to give the house more of an edge. Take a look at the chart below. You will see that the worst cards for the dealer are the 5 and 6, followed closely by the 4. When the dealer is showing a 5 or a 6, they have a 42 percent chance of busting and a 40 percent chance when they have a 4 showing. This is why you double down more often when the dealer is showing a 4, 5, or 6.

I. Probability, Odds for a Blackjack or Natural 21
II. House Edge on Insurance Bet at Blackjack
III. Calculate Double-Down Hands
IV. Calculate Blackjack Pairs: Strict or Mixed Ten-Cards
V. Free Blackjack Resources, Basic Strategy, Casino Gambling Systems

1.1. Calculate Probability (Odds) for a Blackjack or Natural 21

First capture by the WayBack Machine (web.archive.org) Sectember (Sect Month) 1, 2015.

I have seen lots of search strings in the statistics of my Web site related to the probability to get a blackjack (natural 21). This time (November 15, 2012), the request (repeated 5 times) was personal and targeted directly at yours truly:

• 'In the game of blackjack determine the probability of dealing yourself a blackjack (ace face-card or ten) from a single deck. Show how you arrived at your answer. If you are not sure post an idea to get us started!'

Oh, yes, I am very sure! As specified in this eBook, the blackjack hands can be viewed as combinations or arrangements (the order of the elements counts; like in horse racing trifectas).

1) Let's take first the combinations. There are 52 cards in one deck of cards. There are 4 Aces and 16 face-cards and 10s. The blackjack (or natural) can occur only in the first 2 cards. We calculate first all combinations of 52 elements taken 2 at a time: C(52, 2) = (52 * 51) / 2 = 1326.

We combine now each of the 4 Aces with each of the 16 ten-valued cards: 4 * 16 = 64.

The probability to get a blackjack (natural): 64 / 1326 = .0483 = 4.83%.

2) Let's do now the calculations for arrangements. (The combinations are also considered boxed arrangements; i.e. the order of the elements does not count).

We calculate total arrangements for 52 cards taken 2 at a time: A(52, 2) = 52 * 51 = 2652.

In arrangements, the order of the cards is essential. Thus, King + Ace is distinct from Ace + King. Thus, total arrangements of 4 Aces and 16 ten-valued cards: 4 * 16 * 2 = 128.

The odds to get a blackjack (natural) as arrangement: 128 / 2652 = .0483 = 4.83%.

4.83% is equivalent to about 1 in 21 blackjack hands. (No wonder the game is called Twenty-one!)

Calculations for the Number of Cards Left in the Deck, Number of Decks

There were questions regarding the number of cards left in the deck, number of decks, number of players, even the position at the table.

1) The previous probability calculations were based on one deck of cards, at the beginning of the deck (no cards burnt). But we can easily calculate the blackjack (natural) odds for partial decks, provided that we know the number of remaining cards (total), Aces and Ten-Value cards.

Let's take the situation heads-up: One player against the dealer. Suppose that 12 cards were played, including 2 Tens; no Aces out. What is the new probability to get a natural blackjack?

Total cards remaining (R) = 52 - 12 = 40

Aces remaining in the deck (A): 4 - 0 = 4

Ten-Valued cards remaining (T): 16 - 2 = 14

Odds of a natural: (4 * 14) / C(40, 2) = 56 / 780 = 7.2%

(C represents the combination formula; e.g. combinations of 40 taken 2 at a time.)

The probability for a blackjack is higher than at the beginning of a full deck of cards. The odds are exactly the same for both Player and Dealer. But - the advantage goes to the Player! If the Player has the BJ and the Dealer doesn't, the Player is paid 150%. If the Dealer has the blackjack and the Player doesn't, the Player loses 100% of his initial bet!

This situation is valid only for one Player against casino. Also, this situation allows for a higher bet before the round starts. For multiple players, the situation becomes uncontrollable. Everybody at the table receives one card in succession, and then the second card. The bet cannot be increased during the dealing of the cards. Hint: try as much as you can to play heads-up against the Dealer!

The generalized formula is:

Probability of a blackjack: (A * T) / C(R, 2)

A = Aces in the deck

T = Tens in the deck

R = Remaining cards in the deck.

2) How about multiple decks of cards? The calculations are not exactly linear because of the combination formula. For example, 2 decks, (104 cards):

~ the 2-deck case:

C(52, 2) = 1326

C(104, 2) = 5356 (4.04 times larger than total combinations for one deck.)

8 (Aces) * 32 (Tens) = 256

Odds of BJ for 2 decks = 256 / 5356 = 4.78% (a little lower than the one-deck case of 4.83%).

~ the 8-deck case, 416 total cards:

C(52, 2) = 1326

C(416, 2) = 86320 (65.1 times larger than total combinations for one deck.)

32 (Aces) * 128 (Tens) = 4096

Odds of BJ for 8 decks = 4096 / 86320 = 4.75% (a little lower than the two-deck situation and even lower than the one-deck case of 4.83%).

There are NO significant differences regarding the number of decks. If we round the figures, the general odds to get a natural blackjack can be expressed as 4.8%.

The advantage to the blackjack player after cards were played: Not nearly as significant as the one-deck situation.

3) The position at the table is inconsequential for the blackjack player. Only heads-up and one deck of cards make a difference as far the improved odds for a natural are concerned.

• Axiomatic one, let's cover all the bases, as it were. The original question was, exactly, as this: 'Dealing yourself a blackjack (Ace AND Face-card or Ten) from a single deck'. The calculations above are accurate for this unique situation: ONE player dealing cards to himself/herself. The odds of getting a natural blackjack are, undoubtedly, 1 in 21 hands (a hand consisting of exactly 2 cards).
• Such a case is non-existent in real-life gambling, however. There are at least TWO participants in a blackjack game: Dealer and one player. Is the probability for a natural blackjack the same – regardless of number of participants? NOT! The 21 hands (as in probability p = 1 / 21) are equally distributed among multiple game agents (or elements in probability theory). Mathematics — and software — to the rescue! We apply the formula known as exactly M successes in N trials. The best software for the task is known as SuperFormula (also component of the integrated Scientia software package).
• Undoubtedly, your chance to get a natural BJ is higher when playing heads-up against the dealer. The degree of certainty DC decreases with an increase in the number of players at the blackjack table. I did a few calculations: Heads-up (2 elements), 4 players and dealer (5 elements), 7 players and dealer (8 elements).
  • The degree of certainty DC for 2 elements (one player and dealer), one success in 2 trials (2-card hands) is 9.1%; divided by 2 elements: the chance of a natural is 9.1% / 2 = 4.6% = the closest to the 'Dealing yourself a blackjack (Ace AND Face-card or Ten) from a single deck' situation.
  • The chance for 5 elements (4 players and dealer), one success in 5 trials (2-card hands) is 19.6%; distributed among 5 elements, the degree of certainty DC for a blackjack natural is 19.6% / 5 = 3.9%.
  • The probability for 8 elements (7 players and dealer), one success in 8 trials (2-card hands) is 27.1%; equally distributed among 8 elements, the degree of certainty DC of a blackjack natural is 27.1% / 8 = 3.4%.
• That's mathematics and nobody can manufacture extra BJ natural 21 hands... not even the staunchest and thickest card-counting system vendors! The PI... er, pie is small to begin with; the slices get smaller with more mouths at the table. Ever wondered why the casinos only offer alcohol for free — but no pizza?

1.2. Probability, Odds for a Blackjack Playing through a Deck of Cards

The probabilities in the first chapter were calculated for one trial. That is, the odds to get a blackjack in the first two cards. But what are the probabilities to get a natural 21 dealing an entire deck?

1.2.A. Dealing 2-card hands until the deck is dealt entirely

There are 52 cards in the deck. Total number of trials (2-card hands) is 52 / 2 = 26. SuperFormula probability software does the following calculation:

• The probability of at least one success in 26 trials for an event of individual probability p=0.0483 is 72.39%.

1.2.B. Dealing 2-card hands in heads-up play until the deck is dealt entirely

There are 52 cards in the deck. We are now in the simplest real-life situation: heads-up play. There is one player and the dealer in the game. We suppose an average of 6 cards dealt in one round. Total number of trials in this case is equivalent to the number of rounds played. 52 / 6 makes approximately 9 rounds per deck. SuperFormula does the following calculation:

• The probability of at least one success in 9 trials for an event of individual probability p=0.0483 is 35.95%.

You, the player, can expect one blackjack every 3 decks in heads-up play.

2. House Edge on the Insurance Bet at Blackjack

“Insurance, anyone?” you can hear the dealer when her face card is an Ace. Players can choose to insure their hands against a potential dealer's natural

Blackjack Double Down Rule

. The player is allowed to bet half of his initial bet. Is insurance a good side bet in blackjack? What are the odds? What is the house edge for insurance? As in the case of calculating the odds for a natural blackjack, the situation is fluid. The odds and therefore the house edge are proportionately dependent on the amount of 10-valued cards and total remaining cards in the deck.

We can devise precise mathematical formulas based on the Tens remaining in the deck. We know for sure that the casino pays 2 to 1 for a successful insurance (i.e. the dealer does have Ten as her hole card).

We start with the most easily manageable case: One deck of cards, one player, the very beginning of the game. There is a total of 16 Teens in the deck (10, J, Q, K). The dealer has dealt 2 cards to the player and one card to herself that we can see exactly — the face card being an Ace. Therefore, 52 – 3 = 49 cards remaining in the deck. There are 3 possible situations, axiomatic one:

• 1) The player has 2 non-ten cards; there are 16 Teens in the deck = the favorable situations to the player if taking insurance. There are 49 – 16 = 33 unfavorable cards to insurance. However, the 16 favorable cards amount to 32, as the insurance pays 2 to 1. The balance is 33 – 32 = +1 unfavorable situation to the player but favorable to the casino (the + sign indicates a casino edge). In this case, there is a house advantage of 1/49 = 2%.
• 2) The player has 1 Ten and 1 non-ten card; there are 15 Teens remaining in the deck = the favorable situations to the player if taking insurance. There are 49 – 15 = 34 unfavorable cards to insurance. However, the 15 favorable cards amount to 30, as the insurance pays 2 to 1. The balance is 34 – 30 = +4 unfavorable situations to the player but favorable to the casino. In this case, there is a house advantage of 4/49 = 8%.
  • This can be also the case of insuring one's blackjack natural: an 8% disadvantage for the player.
  • This figure of 8% represents the average house edge regarding the insurance bet. I did calculations for various situations — number of decks and number of players.
• 3) The player has 2 Ten-count cards; there are 14 Teens in the deck = the favorable situations to the player if taking insurance. There are 49 – 14 = 35 unfavorable cards to insurance. However, the 14 favorable cards amount to 28, as the insurance pays 2 to 1. The balance is 35 – 28 = +7 unfavorable situations to the player but favorable to the casino. In this case, there is a house advantage of 7/49 = 14%. This is the worst-case scenario: The player should never — ever — even think about insurance with that strong hand of 2 Tens!

Blackjack Double Down Odds Genesis Open

Believe it or not, the insurance can be a really sweet deal if there are multiple players at the blackjack table! Let's say, 5 players, the very beginning of the game. There is a total of 16 Teens in the deck (10, J, Q, K). The dealer has dealt 10 cards to the players and one card to herself that we can see exactly — the face card being an Ace. Therefore, 52 – (10 + 1) = 41 cards remaining in the deck. There are many more possible situations, some very different from the previous scenario:

• 1) The players have 10 non-ten cards; there are still 16 Tens in the deck = the favorable situations to the player if taking insurance. There are 41 – 16 = 25 unfavorable cards to insurance. However, the 16 favorable cards amount to 32, as the insurance pays 2 to 1. The balance is 25 – 32 = –7 favorable situation to the player but unfavorable to the casino (the – sign indicates a player advantage now). In this case, there is a house advantage of 7/41 = –17%. The Player has a whopping 17% advantage if taking insurance in a case like this one!
• 2) The players have 10 Ten-count cards; there are 6 Teens in the deck = the favorable situations to the player if taking insurance. There are 41 – 6 = 35 unfavorable cards to insurance. However, the 6 favorable cards amount to 12, as the insurance pays 2 to 1. The balance is 35 – 12 = +23 unfavorable situations to the player but favorable to the casino. In this case, there is a house advantage of 23/41 = 56%. This is the worst-case scenario: None of the players should ever even think about insurance with those strong hands of 2 Tens per capita!
• 3) Applying the wise aurea mediocritas adagio, there should be an average of 3 or 4 Teens coming out in 11 cards; thus, 12 or 13 Tens remaining in the deck. There are 41 – 13 = 28 unfavorable cards to insurance. However, the 12.5 favorable cards amount to an average of 25, as the insurance pays 2 to 1. The balance is 30 – 25 = +5 unfavorable situations to the player but favorable to the casino. In this case, there is a house advantage of 5/41 = 12%. Unfortunately, even if we consider averages, taking insurance is a repelling bet for the player.

A formula? It would look complicated symbolically, but it is very easy to follow.

HA = {(R – T) – T*2} / R

where —

• HA = house advantage
• R = cards remaining in the deck
• T = Tens remaining in the deck.

• Axiomatic one, buying (taking) insurance can be a favorable bet for all blackjack players, indeed. Of course, under special circumstances — if you see certain amounts of ten-valued cards on the table. The favorable situations are calculated by the formula above.
But, then again, a dealer natural 21 occurs about 5%- of the time — the insurance alone won't turn the blackjack game entirely in your favor.

3. Calculate Blackjack Double-Down Hands

Strictly-axiomatic colleague of mine, writing software leads me into new-ideas territory far more often than not. I discovered something new and intriguing while programming software to calculate the blackjack odds totally mathematically. By that I mean generating all possible elements and distinguishing the favorable elements. After all, the formula for probability is the rapport of favorable cases, F, over total possible cases, N: p = F/N.

Up until yours truly wrote such software, total elements in blackjack (i.e. hands) were obtained via simulation. Problem with simulation is incomplete generation. According to by-now famed Ion Saliu's Probability Paradox, only some 63% of possible elements are generated in a simulation of N random cases.

I tested my software a variable number of card decks and various deck compositions. I noticed that decks with lower proportions of ten-valued cards provided higher percentages of potential double-down hands. It is natural, of course, as Tens are the only cards that cannot contribute to a hand to possibly double down. However, the double-down hands provide the most advantageous situations for blackjack player. Indeed, it sounds like 'heresy' to all followers of the cult or voodoo ritual of card counting!

I rolled up my sleeves and performed comprehensive calculations of blackjack double-downs (2-card hands). The single deck is mostly covered, but the calculations can be extended to any number of decks.

At the beginning of the deck (shoe): Total combinations of 52 cards taken 2 at a time is C(52, 2) = 1326 hands. Possible 2-card combinations that can be double-down hands:

• 9-value cards AND 2-value cards: 4 9s * 4 2s = 16 two-card possibilities
• 8-value cards AND 2-value cards: 4 8s * 4 2s = 16 two-card configurations
• 8-value cards AND 3-value cards: 4 8s * 4 3s = 16 two-card possibilities
• 7-value cards AND 2-value cards: 4 7s * 4 2s = 16 two-card configurations
• 7-value cards AND 3-value cards: 4 7s * 4 3s = 16 two-card possibilities
• 7-value cards AND 4-value cards: 4 7s * 4 4s = 16 two-card configurations
• 6-value cards AND 3-value cards: 4 6s * 4 3s = 16 two-card configurations
• 6-value cards AND 4-value cards: 4 6s * 4 4s = 16 two-card combinations
• 6-value cards AND 5-value cards: 4 6s * 4 5s = 16 two-card possibilities
• 5-value cards AND 4-value cards: 4 5s * 4 4s = 16 two-card combinations
• 5-value cards AND 5-value cards: C(4, 2) = 6 two-card hands (5 + 5 can appear 6 ways).
• Ace AND 2-value cards: 4 As * 4 2s = 16 two-card combinations
• Ace AND 3-value cards: 4 As * 4 3s = 16 two-card possibilities
• Ace AND 4-value cards: 4 As * 4 4s = 16 two-card hands
• Ace AND 5-value cards: 4 As * 4 5s = 16 two-card possibilities
• Ace AND 6-value cards: 4 As * 4 6s = 16 two-card hands
• Ace AND 7-value cards: 4 As * 4 7s = 16 two-card combinations.
• Total possible 2-card hands in doubling down configuration: 262. Not every configuration can be doubled down (e.g. 4+5 against Dealer's 9 or A+2 against 7).
• We look at a double down blackjack basic strategy chart. Some 42% of the hands ought to be doubled-down (strongly recommended): 262 * 0.42 = 110. That figure represents 8% of total possible 2-hand combinations (1362), or a chance equal to once in 12 hands.
• The chance for double-down situations increases with an increase in tens out over the one third cutoff count. The probability for a natural blackjack decreases also — one reason the traditional plus-count systems anathema the negative counts. But what's lost in naturals is gained in double downs — and then some.
• A sui generisblackjack card-counting strategy was devised by yours truly and it beats the traditionalist plus count systems hands down, as it were.
• Be mindful, however, that nothing beats the The Best Casino Gambling Systems: Blackjack, Roulette, Limited Martingale Betting, Progressions. That's the only way to go, the tao of gambling.

4. Calculate Blackjack Pairs: Strict or Mixed Ten-Cards

The odds-calculating software I mentioned above (section III) also counts all possible blackjack pairs. The software, however, considers pairs to be two cards of the same value. In other words, 10, J, Q, K are treated as the same rank (value). My software reports data as this fragment (single deck of cards):

Mixed Pairs: All 10-Valued Cards Taken 2 at a Time

Evidently, there are 13 ranks. Nine ranks (2 to 9 and Ace) consist of 4 cards each (in a single deck). Four ranks (the Tenners) consist of 16 cards. Total of mixed pairs is calculated by the combination formula for every rank. C(4, 2) = 6; 6 * 9 = 54 (for the non-10 cards). The Ten-ranks contribute: C(16, 2) = 120. Total mixed pairs: 54 + 120 = 174. Probability to get a mixed pair: 174 / 1326 = 13%.

Strict Pairs: Only 10+10, J+J, Q+Q, K+K

But for the purpose of splitting pairs, most casinos don't legitimize 10+J, or Q+K, or 10+Q, for example, as pairs. Only 10+10, J+J, Q+Q, K+K are accepted as pairs. Allow me to call them strict pairs, as opposed to the above mixed pairs.

There are 13 ranks of 4 cards each. Each rank contributes C(4, 2) = 6 pairs. Total strict pairs: 13 * 6 = 78. Probability to get a mixed pair: 78 / 1326 = 5.9%.Total strict pairs = 78 2-card hands (5.9%, but...).

However, not all blackjack pairs should be split; e.g. 10+10 or 5+5 should not be split, but stood on or doubled down. Only around 3% of strict pairs should be legitimately split. See the optimal split pairsblack jack strategy card.

5. Free Blackjack Resources, Basic Strategy, Casino Gambling Systems

• Blackjack Mathematics Probability Odds Basic Strategy Tables Charts.
• The Best Blackjack Basic Strategy: Free Cards, Charts.
  ~ All playing decisions on one page — absolutely the best method of learning Blackjack Basic Strategy (BBS) quickly (guaranteed and also free!)
• Blackjack Gambling System Based on Mathematics of Streaks.
• Blackjack Card Counting Cult, Deception in Gambling Systems.
• The Best Blackjack Strategy, System Tested with the Best Blackjack Software.
• Reality Blackjack: Real, Fake Odds, House Advantage, Edge.

Back to Forums IndexSocrates HomeSearch

Blackjack is one of the casino games with the lowest possible house edge, which can be attributed to its favourable set of rules. One such rule that works to the benefit of players is the double down option. It enables you to increase your initial bet when you find yourself in advantageous situations.

Doubling down can be both exciting and profitable for the player provided that they know when to make this move correctly. The situations when this play becomes optimal do not occur all that often at the blackjack table. One must learn to identify them in order to extract the most value from doubling their wagers.

• £10Minimum Deposit
• Mobile Casino
• 24/7 Support

• £10Minimum Deposit
• Mobile Casino
• 24/7 Support

• £20Minimum Deposit
• Mobile Casino
• 24/7 Support

Regrettably, many blackjack players do not recognize the correct spots that call for a double down and end up losing tons of money as a result. In this article, GamblingPlex expands on what doubling down is in blackjack, provides readers with some strategy hints for this move, and explains why you should never double down for less than your original wager.

How to Double Down in Blackjack

To begin with, doubling is available to players only on their starting hands before they have drawn any more cards to their initial totals. When a player wishes to double their bet in the game of blackjack after their hand has been dealt, they can do so by signalling they want a double down. The hand signal used in shoe games differs from that in single-deck and double-deck variations.

In multi-deck blackjack where the cards are dealt out of a shoe, you double down by posting an additional wager up to the amount of your original wager and pointing towards your betting box. You place the extra chips in your betting box next to your initial bet but never on top of it.

This is for the benefit of the dealer, allowing them to easily distinguish your double down wager from your original bet. The dealer will then draw no more than one card to your starting hand. The third card is usually placed perpendicularly, next to the first two cards, to indicate this hand has been doubled on. There will be no more cards dealt to that particular hand.

When playing single-deck and double-deck blackjack, you double down by making an extra bet and placing your first two cards face-up on the layout. The dealer would then tuck the third card under your chips.

The third card is dealt face-down in this case and is exposed after the dealer has finished playing their hand. This further adds to the excitement of doubling, especially when the dealer busts. With that said, players in hand-held games are permitted to peek under the double-down card to see its value but should do so without exposing it. The profits or losses you register during this round are based on the new overall wager.

For example, if you had initially wagered $10 and have doubled for the full amount of $10, you now have a total of $20 in action and will win $20 on top of that, for an overall payout of $40. If the dealer beats you, you will lose $20 rather than $10 only.

Double Down Blackjack Meaning

The OBO Rule

This is not the case in many European variations where no hole cards are in play. The dealer receives one exposed card during the initial deal and does not draw a second one until after all patrons have finished playing their hands.

Such games usually implement the so-called “original bets only” rule, abbreviated as OBO. Whenever the dealer hits a blackjack, players would lose only their initial wagers under this rule. Additional bets made on doubling and splitting push and are returned to players.

Choosing a Blackjack Table

Doubling is an interesting choice, and it could pay quite well when done properly. However, you should not forget to check the specific rules that apply to doubling at your chosen table before you sit down. Blackjack is infamous throughout the gambling world for its diversity when it comes to rules. Playing conditions would often vary not only between different casinos but between different blackjack tables within the same gaming venue.

Before you sit down, check whether you can double on any two cards or just on hands with totals of 9, 10, and 11. When restricted to doubling only on these three hands, players suffer a house edge increase of 0.09%. Some tables are even more restrictive, allowing you to make this play only on two-card totals of 10 and 11, which adds 0.18% to the casino advantage.

Another thing worth checking beforehand is whether the table supports doubling after a split (DAS). The absence of DAS also leads to an increase in the house edge, this time by 0.14%.

Keep in mind that the exact doubling rules, applicable at a given table, are not displayed on the layout as is the case with the dealer’s standing total and the blackjack payouts. We suggest you ask the dealer or the pit boss before you take a seat if you are unsure whether you can double on any two-card hand.

When Should You Double Down?

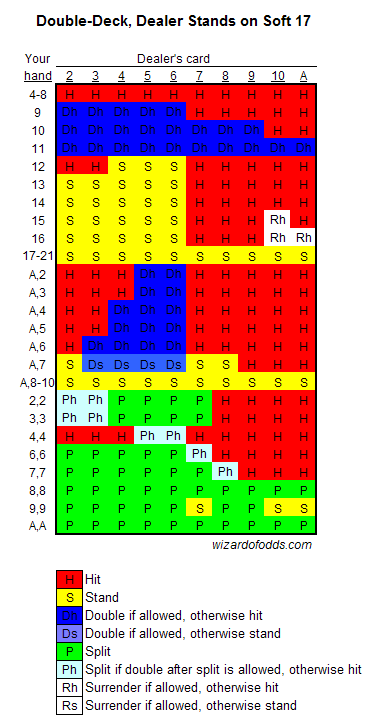
New players usually rely more on their hunches and intuition when doubling, which often leads to bad decision-making and unnecessary frustration. As exciting as it is, doubling is not the optimal move at all times. This play is recommended only on specific two-card hands against certain upcards that put the dealer in a weak spot.

It makes no sense to increase your wager against a dealer who shows strong upcards, if you, yourself, have a bad starting total like 12 or 15, for example. One situation where doubling down has been proven to show the best results is when a player is holding a total of 11 against a dealer with a 6. The probability of the dealer busting with this upcard is a little over 42%.

Meanwhile, your chances of prevailing with a total of 11 against the 6 are inevitably higher than 50% regardless of the exact composition of your hand as you can see below. Doubling down when the odds are on your side will have an overall positive effect on your profitability. The figures correspond to a six-deck S17 game where the peek rule is in place.

The Probabilities for a Player Total of 11 against the Dealer’s 6

Player Hand Composition	Probability of Winning	Probability of Pushing	Probability of Losing
6/5 vs. 6	63.80%	6.60%	29.60%
7/4 vs. 6	63.60%	6.70%	29.70%
8/3 vs. 6	63.50%	6.70%	29.80%
9/2 vs. 6	63.50%	6.70%	29.90%

The optimal plays for doubling are covered by basic strategy, which is presented in the form of a chart. You can start seeing more profits from your double downs if you stick to basic strategy. Feel free to consult with your strategy card when playing online or at a landbased casino until you learn all the correct moves by heart.

Doubling on Hard Hands

Before we proceed any further with strategy hints, we would like to specify that the correct doubling moves may differ, depending on whether you have a hard or a soft total. A hard hand is one that either lacks an ace or has one, but its value is 1 only.

Doubling on hard totals of 12 or higher is never recommended since your chances of busting are greater and you risk losing twice as much money. The higher the hard total, the higher your chances of going over 21 by drawing a third card.

You can find the correct moves for doubling below but keep in mind they correspond to six-deck games with DAS under the S17 rule for the dealer. Some of the playing decisions are different when you double against a dealer who hits soft 17 (H17). Certain moves also vary based on deck number. Refrain from using multi-deck strategy cards for single-deck and double-deck blackjack to prevent potential playing errors.

• Double down on hard 9 when the dealer’s exposed card is 3, 4, 5, or 6.
• Double down on hard 10 against all dealer upcards except for 10 and ace. This applies also when you get dealt a pair of 5/5, which you should never split. You double down on your 5/5 against dealer upcards 2 through 9, instead.
• Double down on hard 11 against all exposed cards of the dealer except for the ace. On a side note, players are recommended to double even against the dealer’s ace in shoe-dealt H17 blackjack.

Doubling on Soft Hands

Soft hands call for a different approach because they contain aces whose value fluctuates. The aces can be assigned a value of either 1 or 11, based on the holder’s preferences. You have more chances of making successful doubles with such hands because the ace’s flexibility prevents you from going over 21 by taking one more card from the dealer.

The worst that could happen is for you to get dealt a small-value card and end up with a low hand total. It is also possible to catch a ten-value card and transform your hand from a soft one into a hard one.

Here is an example. You have A/5, or soft 16, which you can treat as either 6 or 16. The dealer’s upcard is a 6 which calls for soft doubling. The dealer pulls out a King for your double down and you get stuck with a hard 16. Check out the plays recommended below. They are again optimal in multiple-deck blackjack where the dealer is required to stand on soft 17 (S17).

• Soft 13 (A/2) and soft 14 (A/3) require a double down when the dealer’s exposed card is 5 or a 6.
• Soft 15 (A/4) and soft 16 (A/5) call for a double when the dealer shows a 4, 5, or 6.
• Soft 17 (A/6) and soft 18 (A/7) require a double when the dealer’s upcard is 3, 4, 5, or 6. In H17 games, you double on soft 18 against the dealer’s deuce as well. Soft 19 (A/8) also calls for a double down against the dealer’s 6 in H17 games.

Should You Double for Less?

As we told you earlier, you can double down for an amount up to your original wager although no one prohibits you from doubling for less than what you have initially bet. For instance, if you have originally wagered $20, you can double for $15, $10 or even $5.

In fact, this is possible in almost all landbased casinos. However, you must always double for the full amount when playing online randomly generated variations of blackjack. Many rookies or players with tight bankrolls prefer to double for less, mainly because they feel uncomfortable with exposing more money at risk by covering their initial wagers in full. Others skip on doubling altogether by hitting or standing, both of which do not require them to invest more money.

How Doubling Compares to Hitting

It is no coincidence doubling down is recommended over hitting and standing in certain cases. You are entitled to no more than one card when doubling, which is why you sometimes get stuck with bad stiff hands like hard 15 or hard 14.

Blackjack Double Down Odds Explained

Since it is impossible to hit after a double down, the percentage of the times when you beat the dealer with doubling is smaller than that for hitting. However, the small difference in win rates is offset by the larger profits you receive for successful doubling.

Suppose, for instance, you receive a total of 11 against a dealer with a ten-value card. As you can see from the strategy hints, this situation calls for a double down in shoe games with the S17 rule. Your 11 will win against the ten-value card 56 per every 100 hands if you hit and 54 per every 100 hands if you double down.

Respectively, you will have 54 x $20 = $1,080 in earnings, if you double for the full amount on a $10 initial wager. Your losses with 11 against the dealer’s ten-value card will be 46 x $20 = $920 on average. You will win approximately $160 every hundred hands in the long term.

Meanwhile, your earnings when you hit your 11 against the dealer’s ten will amount to 56 x $10 = $560 whereas your losses will be 44 x $10 = $440. Thus, hitting yields net profits of $120. This leads us to the logical conclusion doubling on 11 against the dealer’s 10 is better for the player than hitting because it yields $40 more in profits per every hundred hands on average.

Doubling for Less vs. Doubling for the Full Wager

Similarly to hitting your 11 against the dealer’s ten, doubling for less on this hand is nonsensical from a basic-strategy perspective. The whole purpose of doubling is to enable the player to boost their gains when in favourable situations. This is impossible unless you double down for your full wager.

This becomes blatantly obvious when we continue with our example where your main bet is $10. Only this time, you choose to double for $5 only instead of hitting. Your average earnings per one hundred hands will be equal to 54 x $15 = $810 whereas your average losses will be 46 x $15 = $690 if OBO is not in place.

This makes for overall net winnings of $120, which is again $40 less than the average earnings you will register when you double for the full amount. This sum will drop further down if you are a scrape enough to double down for one-fourth of your initial $10 bet, or $2.50.

In this scenario, your average wins per one hundred hands will amount to 54 x $12.50 = $675 whereas your losses will add up approximately to 46 x $12.50 = $575. Your total of 11 will earn you only $100 against the dealer’s ten in the long run.

Blackjack Double Down Odds Poker

It is easy to see why doubling for the full amount is the best course of action, compared to hitting or doubling for less. This applies to all correct doubling plays, not only to a total of 11 against a ten.

More Articles Covering Blackjack

Blackjack Odds Sheet

Blackjack Basics
Blackjack Rules
Blackjack Variations
Multi Hand Blackjack
Single Deck Blackjack Rules
Vegas Downtown Blackjack
Vegas Strip Blackjack Rules
Atlantic City Blackjack
How to Hit a Hand
Splitting Hands in Blackjack
Insurance in Blackjack
Surrender in Blackjack
Standing a Hand
Blackjack Double Down
Splitting Hands in Blackjack
Blackjack Odds
Blackjack Strategy for Playing Hands
Blackjack Cards and Hands Value

Blackjack Double Down Odds Video Poker